

Furthermore, we know that the more massive the door is, the more slowly it opens this is because angular acceleration is inversely proportional to mass. For example, a door opens slowly if you push too close to its hinge, but opens easily if you push far from the hinges. The farther the force is applied from the pivot point (or fulcrum), the greater the angular acceleration. If you have ever spun a bike wheel or pushed a merry-go-round, you know that force is needed to change angular velocity. They blow houses away as if they were made of paper and have been known to pierce tree trunks with pieces of straw. Tornadoes produce wind speeds as high as 500 km/h (approximately 300 miles/h), particularly at the bottom where the funnel is narrowest because the rate of rotation increases as the radius decreases. The difference in wind speeds between the strong cold winds higher up in the atmosphere in the jet stream and weaker winds traveling north from the Gulf of Mexico causes the axis of the column of rotating air to shift as the storm travels so that the axis becomes vertical, creating a tornado. They come out of severe thunderstorms called supercells, which have a column of air rotating around a horizontal axis, usually about four miles across. Tornadoes are perfect examples of rotational motion in action in nature. The most active part of the world for tornadoes, called tornado alley, is in the central United States, between the Rocky Mountains and Appalachian Mountains. Since storm chasers follow in the wake of the destruction left by tornadoes, changing flat tires due to debris left on the highway is common. Storm chasing is a dangerous pastime because tornadoes can change course rapidly with little warning. Storm chasers tend to fall into one of three groups: Amateurs chasing tornadoes as a hobby, atmospheric scientists gathering data for research, weather watchers for news media, or scientists having fun under the guise of work. National Oceanic and Atmospheric Administration) These equations can be used to solve rotational or linear kinematics problem in which a and α α are constant.įigure 6.10 Tornadoes descend from clouds in funnel-like shapes that spin violently. In fact, all of the linear kinematics equations have rotational analogs, which are given in Table 6.3. Notice that the equation is identical to the linear version, except with angular analogs of the linear variables. Where ω 0 ω 0 is the initial angular velocity. The equation for the kinematics relationship between ω ω, α α, and t is Recall the kinematics equation for linear motion: v = v 0 + a t v = v 0 + a t (constant a).Īs in linear kinematics, we assume a is constant, which means that angular acceleration α α is also a constant, because a = r α a = r α. It only describes motion-it does not include any forces or masses that may affect rotation (these are part of dynamics). The kinematics of rotational motion describes the relationships between the angle of rotation, angular velocity, angular acceleration, and time.

In the case of linear motion, if an object starts at rest and undergoes a large linear acceleration, then it has a large final velocity and will have traveled a large distance. Putting this in terms of the variables, if the wheel’s angular acceleration α α is large for a long period of time t, then the final angular velocity ω ω and angle of rotation θ θ are large. For example, if a motorcycle wheel that starts at rest has a large angular acceleration for a fairly long time, it ends up spinning rapidly and rotates through many revolutions. We can now begin to see how rotational quantities like θ θ, ω ω, and α α are related to each other. Table 6.2 Rotational and Linear Variables The student knows and applies the laws governing motion in a variety of situations.
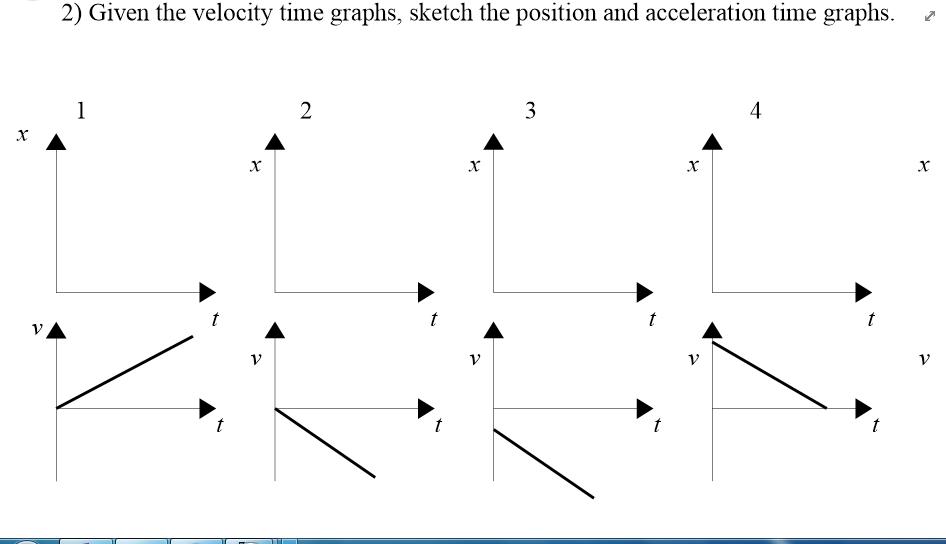
Unit 3 stacks of kinematics curves manual#
In addition, the High School Physics Laboratory Manual addresses content in this section in the lab titled: Circular and Rotational Motion, as well as the following standards:


 0 kommentar(er)
0 kommentar(er)
